Optical conveyor belt

I. Easy-physics introduction

We tested an optical tool that enables precise delivery of submicron particles over a distance of hundreds of micrometers. We employed two counter propagating laser beams as a lace and we string submicron beads on it. To obtain this laser lace as long as possible but as narrow as possible we used special type of laser beams – so called Bessel beams. These beams exist over very long distance without changing their properties (see e.g. work of Prof. Dholakia's optical trapping group in St. Andrews or Prof. Bouchal's group). In spite of its unique properties it can be generated quite easily using an optical element called axicon (glass cone).

But only this would not enable controlled bead delivery, we needed knots on the lace. Again we used an optical tool and we let the beams interfere and a periodic array of intensity maxima (antinodes) and minima (nodes) was created – so called standing wave. The distance between the knots (intensity maxima) is very small, about ½ of the wavelength of the laser light.

If the beads are inserted into the cuvette with liquid, they tend to be caught at these intensity maxima (or minima – according to bead size). If we were able to move with the knots with caught beads we could deliver them as will. Even here optics can be employed. If the phase of one of the interfering beam is changed, the whole structure of intensity maxima and minima (the whole standing wave) is moved along the axis of both beams.

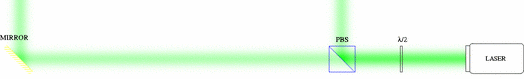In co-operation with colleagues from University of St. Andrews we set-up an experimental device that used fiber laser with maximal used power 4W (the wavelength was 1070 nm). We tested small polystyrene particles of diameters 410, 490, 600 800 930 and 1000 nm. We observed such tight objects perpendicularly to the beam propagation via the photons that were scattered by these beads to CCD camera (we have not used illumination).

Bessel beam has other very useful property – it can self-heal behind obstacle and restore its original shape that had before the obstacle. This property perfectly fits our application since many objects can be caught and they do not disturb or spoil the beam properties.

See also AIP Physics News, About physics, or Physics Today, Vol. 58, No. 7, 2005: Physics Update 

The detailed set-up was the following:

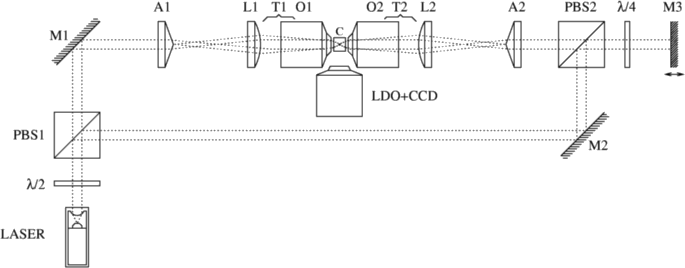
• As a source of coherent light we used a fiber laser (IPG Photonics TLR-series) working at λ=1.070 μm with maximum power of 10 W.

• The linearly polarized beam coming out of the fiber was divided by a polarization beam-splitter PBS1 into two paths and the power ratio between them was set by a half-wave plate λ/2.

• The first p-polarized beam is reflected on metallic mirror M1 and transformed to the BB by an axicon A1 (Eksma 130-0270, 170^o apex angle and 1 inch in diameter). The core of this BB is decreased by a telescope T1 consisted of a lens L1 (f=38mm) and microscope objective O1 (Newport, M-40x, N.A. 0.40). Afterwards the BB is sent to a cuvette 5 mm long made from 1 mm thick BK7 glass. It was filled up with D₂O which has lower absorption at the trapping wavelength and so the heating and unwanted convection of the liquid was suppressed. From the parameters of the optical system we obtained that the diameter of the central BB core is equal to 2.24 μm.

• The second s-polarized beam is after reflection on the metallic mirror M2 delivered on a polarization beam-splitter PBS2. Here it is reflected, passed through the quarter-wave λ/4 plate to the axially movable mirror M3 controlled by stepmotor (Newport ESP 300. After reflection back through the same λ/4 the beam became p-polarized, propagated through the PBS2 to axicon A2 (Eksma 130-0260, 160^o apex angle and 1 inch in diameter) and was transformed to the BB. The telescope T2 decreased the width of the BB central core to 2.14 μm and consisted of a lens L2 (f=38mm) and microscope objective O2 (Newport, M-20x, N.A. 0.40). Even though we had not identical optical components in both paths at the time of this experiment, the estimated widths of both counter-propagating beams are very close to each other.

• The beams interfered in the cuvette and the distance between two successive antinodes is Δ z = λ_vac /[n_D2O ( cos(psi₁) +cos(psi₂) )] , where ψ₁ (ψ₂) is the angle that makes plane wave k vectors forming the first (second) BB with propagation z axis. In our case we obtained δ z=419 nm which is slightly bigger comparing to counter-propagating plane waves δ z =λ_vac /(2 n_D2O)=402 nm.

• The microparticles were put into the cuvette and scattered light was imaged on a CCD camera by a microscope objective LDO with long working distance (Mitutoyo, MPlan NR50 0.42, f=200).

The Bessel beam has intensity maxima side-lobes. Fortunately in our case they were much smaller than the central ones.

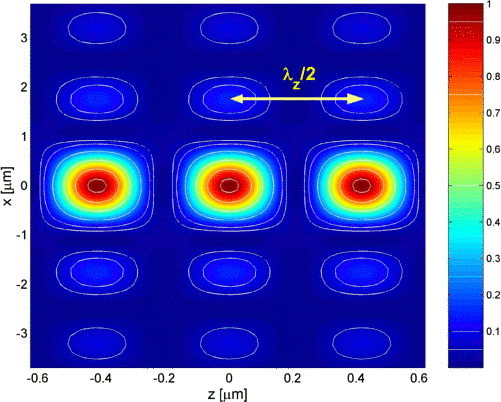
Theoretical simulations revealed that certain particle sizes could not be confined since they "do not feel" the standing wave.

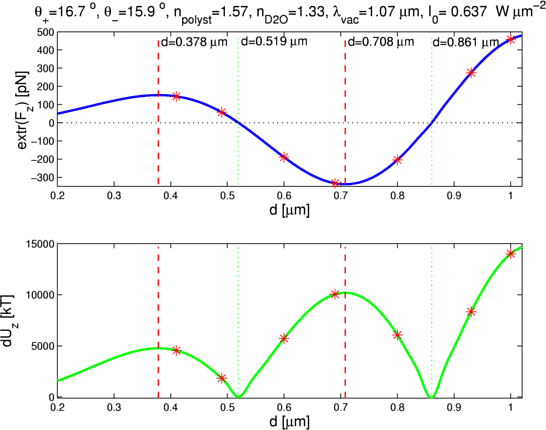
The theoretical dependence of the extremal (maximal or minimal) axial optical force (top plot) and trap depths δU_z (bottom plot) in the BSW as a function of polystyrene beads diameter. Particles were confined in D₂O using on-axis optical intensity I₀=0.637 W / μm^-2 in each beam (equivalent to on-axis intensity in Gaussian beam of beam waist 1 μm and power 1 W) of the idealized Bessel beam created by the same optics as in the experiment. The negative values of extremal forces indicates that the bead is trapped with its centre at node of the standing wave. Stars denote the beads used in experiments. Vertical dotted lines indicate bead sizes that do not feel the BSW and therefore cannot be confined in this periodic structure.

In order to compare theory with experimental results more precisely, we applied correlation algorithm to track the particles trajectory along both the x and z axis from the video records. The results shown in the insets of figure above support the results that smaller sphere is strongly confined since it stayed in one trap. On the other hand plenty of jumps between neighboring longitudinal traps occurred for bigger sphere and the distances between them fit very well to the distance between two neighboring SW antinodes δz=419 nm. Unfortunately this experimental system was not stable enough and the small drift of the longitudinal traps smeared the clear distinction between neighboring traps during repeated particle returns to the same trap.

We calculated the trap stiffness from the time record of x and z bead positions. We choose ranges of the same number of consecutive positions (50), eliminated the drift by fitting a line and calculated root-mean-square deviations (RMSD) <x²> and <z²> from this line. The trap stiffness is inversely proportional to the RMSD. Unfortunately we could not compare the experimental stiffness with theoretical predictions because we did not know the exact trapping laser intensity at the place of particle confinement. This quantity is not experimentally available since the ideal BB is in reality longitudinally modulated by Gaussian envelope and so it is dependent on the axial position of the bead. For this reason during the exchange of the bead sizes we tried to put the cuvette to the same places and kept the same laser power. Nevertheless we could compare the ratio of stiffnesses κ_z/κ_x=<x²>/<z²>, that is insensitive to trapping power.

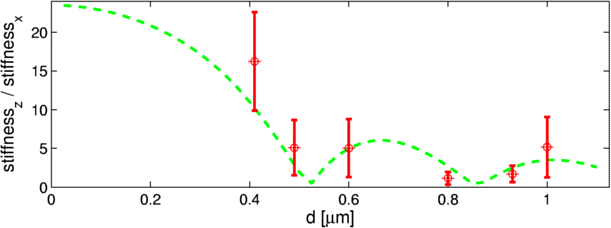
We believe that this comparison reflects the theoretical trends even though the error bars indicate that much more attention must be paid to the stability of the system in future measurements. The ratio is bigger than 2 for the majority of studied particle diameters and therefore the objects are confined tighter longitudinally than laterally. In contrast for a single focused GB where this ratio lies between 0.68 for d=400 nm and 0.2 for d=1000 nm , respectively.

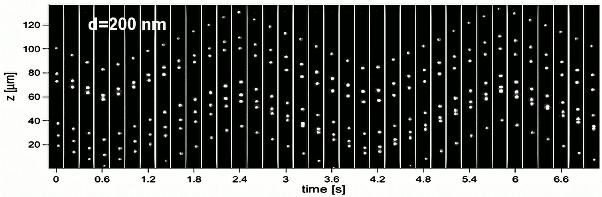
With other set-up where we focused on two identical optical paths and we used different laser of wavelength 532 nm we succeeded in delivery of even smaller objects of diameter 200 nm. Also the total available delivery distance was close to 1 mm.

More you can find in our related publications

T. Cizmar, M. Siler, P. Zemanek: "An optical nanotrap array movable over a milimetre range",
       Appl. Phys. B 84, 197-203, 2006,   ABSTRACT   DOWNLOAD

T. Cizmar, V. Garces-Chavez, K. Dholakia, P. Zemanek: "Optical conveyor belt for delivery of submicron objects",
       Appl Phys Lett 86, 174101-1, 2005,   ABSTRACT   DOWNLOAD

T. Cizmar, V. Garces-Chavez, K. Dholakia, P. Zemanek: "Optical conveyor belt for delivery of sub-micron objects",
       Proceedings of SPIE 6180, 618027:1-7, 2006, Photonics, Devices and Systems III, Praha(CZ), 8-11 June 2005,   ABSTRACT

T. Cizmar, V. Garces-Chavez, K. Dholakia, and P. Zemanek: "Optical conveyor belt based on Bessel beams",
       Proceedings of SPIE 5930, 0X1-0X7, 2005,   ABSTRACT   DOWNLOAD

T. Cizmar, V. Garces-Chavez, K. Dholakia, P. Zemanek: "Optical trapping in counter-propagating Bessel beams",
       Proceedings of SPIE 5514, 643-651, 2004,   ABSTRACT   DOWNLOAD

Send comments to webmaster
Last modification: 29 Jul 2007